Real Trigonometry

Updated May 2018
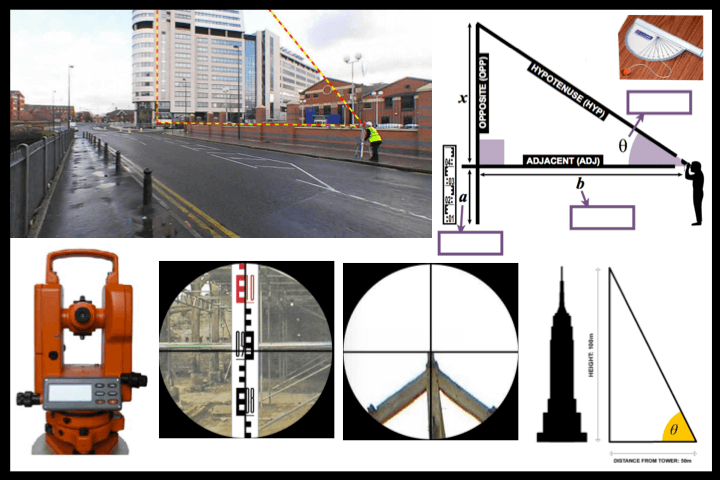
Trigonometry: use in finding heights
These excellent materials were designed at Leeds Metropolitan University and are excellent for seeing trigonometry in real use.
- Teacher/pupil task sheet with Clinometer template for angle measuring.
- Theodolite poster – how to use to find a height.
- File showing how to use theodolite: 15 pages includes answers.
- Another theodolite example to find height of a wall.
- The related YouTube channel (here) has 5 videos including one that shows how to make the clinometer (here).
- Instructions and template for making a trig-wheel.
Trigonometry: ship’s course
Another example of a real use of trigonometry (to find a ship’s course) comes from Maths at Work, on the NCETM site (found here): includes video.
Trigonometry: height of tower at British Museum
The British Museum has a KS3 challenge to find the height of a tower in its great court (February 2016). BM KS3 Maths Challenge (includes other ideas as well).
Practical lesson
- Create clinometers or borrow theodolites. Borrow long tapes from PE, use metre rules or laser distance measures.
- Go outside and have pupils stand different distances away from eg the school building (if you can).
- Measure distance from school building, use clinometer to find angle, take distance of height of clinometer above ground level.
- Have each pupil calculate height of building and then use mean, mode or median to decide ‘height’.
- Consider rounding, accuracy, think about which errors of measurement have the biggest impact.
- Think about other ways to find the height of the building – could any of these be more accurate?
- Find true height of building from building plans!
- With brick buildings finding the height of e.g. 10 layers of bricks is usually the most accurate method.
Other reources
- 33 page Trigonometry resource book found here.
- Jo Morgan’s Resourceaholic ideas here.
- Don Steward’s Median resources here and exact values for trigonometry here.
MEP Resources for Trigonometry
- Pupil Text
- Practice Book
- Further Practice Book
- Answers to Pupil Text – Trig start on page 22 shown as page 272